H2 Standard Enthalpy Of Formation

5.7: Enthalpies of Formation

Last updated
Save as PDF

Page ID: 39100

Skills to Develop

to understand Enthalpies of Formation and be able to apply them to calculated Enthalpies of Reaction

Enthalpies of Formation

One way to report the heat absorbed or released would exist to compile a massive fix of reference tables that list the enthalpy changes for all possible chemic reactions, which would crave an incredible amount of effort. Fortunately, Hess's law allows us to calculate the enthalpy change for near any conceivable chemical reaction using a relatively small ready of tabulated information starting from the elemental forms of each atom at 25 ^oC and 1 atm pressure.

Enthalpy of formation (ΔH _f): The enthalpy change for the germination of i mol of a chemical compound from its component elements, such as the germination of carbon dioxide from carbon and oxygen. The corresponding relationship is

\[ elements \rightarrow chemical compound \;\;\;\;\ \Delta H_{rxn} = \Delta H_{f} \tag{5.seven.one} \]

For example, consider the combustion of carbon
\[ C_{(s)} + O_{2 (g)} \rightarrow CO_{ii}\left (g \right ) \; \; \; \; \Delta H_{rxn} = \Delta H_{f}\left [CO_{ii}\left ( g \correct ) \right ] \]
The sign convention for ΔH _f is the same as for any enthalpy change: ΔH _f < 0 if heat is released when elements combine to form a compound and ΔH _f > 0 if oestrus is absorbed.

Note

The sign convention is the same for all enthalpy changes: negative if heat is released by the organisation and positive if heat is absorbed by the system.

Standard Enthalpies of Formation

The magnitude of ΔH for a reaction depends on the physical states of the reactants and the products (gas, liquid, solid, or solution), the pressure of any gases present, and the temperature at which the reaction is carried out. To avoid defoliation caused by differences in reaction conditions and ensure uniformity of data, the scientific customs has selected a specific set of weather under which enthalpy changes are measured. These standard conditions serve equally a reference signal for measuring differences in enthalpy, much as sea level is the reference point for measuring the height of a mountain or for reporting the distance of an aeroplane.

The standard weather: 1 atm for all gases and a concentration of 1.0 M for all species in solution. for which most thermochemical data are tabulated are a pressure level of 1 atmosphere (atm) for all gases and a concentration of 1 One thousand for all species in solution (1 mol/L). In addition, each pure substance must be in its standard state, which is commonly its most stable form at a pressure of 1 atm at a specified temperature. We assume a temperature of 25°C (298 Yard) for all enthalpy changes given in this text, unless otherwise indicated. Enthalpies of formation measured under these conditions are chosen standard enthalpies of formation (ΔH^o _f ) The enthalpy change for the formation of 1 mol of a compound from its component elements when the component elements are each in their standard states. The standard enthalpy of formation of any element in its most stable class is zero past definition. (which is pronounced "delta H eff naught"). The standard enthalpy of formation of any element in its standard state is zip by definition. For example, although oxygen can exist every bit ozone (O₃), atomic oxygen (O), and molecular oxygen (O_two), O₂ is the most stable class at 1 atm pressure and 25°C. Similarly, hydrogen is H₂(g), not atomic hydrogen (H). Graphite and diamond are both forms of elemental carbon, merely because graphite is more stable at 1 atm pressure and 25°C, the standard country of carbon is graphite (Figure nine.7.one). Therefore, O₂(g), H₂(one thousand), and graphite have ΔH^o _f values of zip.

Effigy 9.7.1 Elemental Carbon. Although graphite and diamond are both forms of elemental carbon, graphite is more stable at 1 atm pressure and 25°C than diamond is. Given plenty time, diamond will revert to graphite under these atmospheric condition. Hence graphite is the standard state of carbon.

The standard enthalpy of formation of glucose from the elements at 25°C is the enthalpy alter for the following reaction:

\[ 6C\left (s, graphite \right ) + 6H_{2}\left (g \correct ) + 3O_{2}\left (g \right ) \rightarrow C_{6}H_{12}O_{half-dozen}\left (s \right )\; \; \; \Delta H_{f}^{o} = - 1273.3 \; kJ \tag{5.7.2} \]

It is not possible to measure the value of ΔH^o _f for glucose, −1273.3 kJ/mol, by only mixing appropriate amounts of graphite, O_ii, and H₂ and measuring the heat evolved as glucose is formed; the reaction shown in Equation 9.7.2 does not occur at a measurable rate under any known atmospheric condition. Glucose is not unique; about compounds cannot be prepared by the chemical equations that define their standard enthalpies of formation. Instead, values of are obtained using Hess's law and standard enthalpy changes that accept been measured for other reactions, such every bit combustion reactions. Values of Δ H ^o _f for an extensive list of compounds are given in Appendix A. Note that Δ H ^o _f values are always reported in kilojoules per mole of the substance of interest. Also detect in Appendix A that the standard enthalpy of formation of O₂(g) is zero because information technology is the most stable form of oxygen in its standard state.

Example 5.7.ane

For the formation of each compound, write a balanced chemical equation corresponding to the standard enthalpy of formation of each compound.

HCl(one thousand)
MgCO₃(s)
CH_iii(CH₂)₁₄CO₂H(s) (palmitic acrid)

Given: compound

Asked for: balanced chemical equation for its formation from elements in standard states

Strategy:

Use Appendix A to identify the standard state for each element. Write a chemical equation that describes the formation of the chemical compound from the elements in their standard states and so residual it so that 1 mol of product is made.

Solution:

To calculate the standard enthalpy of formation of a chemical compound, we must start with the elements in their standard states. The standard state of an element can be identified in Appendix A: by a ΔH^o _f value of 0 kJ/mol.

Hydrogen chloride contains one atom of hydrogen and 1 atom of chlorine. Because the standard states of elemental hydrogen and elemental chlorine are H_ii(g) and Cl₂(g), respectively, the unbalanced chemical equation is
\[H _{2(g)} + Cl_{ii(g)} \rightarrow HCl_{(thousand)}\]
Fractional coefficients are required in this case considering ΔH ^o _f values are reported for 1 mol of the product, HCl. Multiplying both H₂(g) and Cl₂(g) by i/2 balances the equation:
\[ \dfrac{i}{2}H_{ii}\left ( yard \right )+ \dfrac{one}{2}Cl_{2}\left ( g \right ) \rightarrow HCl\left ( g \correct ) \]
The standard states of the elements in this chemical compound are Mg(s), C(s, graphite), and O_two(one thousand). The unbalanced chemical equation is thus
\[Mg_{(s)} + C_{(south, graphite)} + O_{₂(g)} \rightarrow MgCO₃(southward)\]
This equation can exist balanced past inspection to give
\[ Mg\left ( one thousand \correct )+ C\left ( south, graphite \right ) \frac{3}{2}O_{2}\left ( g \right )\rightarrow MgCO_{3}\left ( s \correct ) \]
Palmitic acid, the major fatty in meat and dairy products, contains hydrogen, carbon, and oxygen, then the unbalanced chemical equation for its formation from the elements in their standard states is every bit follows:
C(s, graphite) + H_two(yard) + O_ii(m) → CH_three(CH_two)_fourteenCO_iiH(s)
There are sixteen carbon atoms and 32 hydrogen atoms in ane mol of palmitic acrid, so the balanced chemical equation is
16C(s, graphite) + 16H₂(g) + O₂(m) → CH₃(CH₂)_xivCO_twoH(s)

Exercise five.7.i

For the formation of each compound, write a balanced chemical equation corresponding to the standard enthalpy of formation of each compound.

NaCl(s)
H_iiSO₄(fifty)
CH₃CO_iiH(50) (acetic acrid)

Reply:

\( Na\left ( g \right )+ \frac{one}{two}Cl_{2}\left ( g \right )\rightarrow NaCl\left ( due south \correct ) \)
\( H_{2}\left ( g \right ) + \frac{one}{8}S_{viii}\left ( due south \right ) + 2O_{2}\left ( g \right ) \rightarrow H_{2}SO_{4}\left ( fifty \right ) \)
2C(s) + O_two(one thousand) + 2H₂(g) → CH₃CO_iiH(l)

Standard Enthalpies of Reaction

Tabulated values of standard enthalpies of germination can be used to calculate enthalpy changes for any reaction involving substances whose \(\Delta{H_f^o}\) values are known. The standard enthalpy of reaction (ΔH_rxn ) is the enthalpy modify that occurs when a reaction is carried out with all reactants and products in their standard states. Consider the general reaction

\[ aA + bB \rightarrow cC + dD \tag{v.7.3}\]

where A, B, C, and D are chemical substances and a, b, c, and d are their stoichiometric coefficients. The magnitude of ΔH^ο is the sum of the standard enthalpies of formation of the products, each multiplied by its advisable coefficient, minus the sum of the standard enthalpies of formation of the reactants, likewise multiplied by their coefficients:

\[ \begin{matrix} \Delta H_{rxn}^{o} = \left [c\Delta H_{f}^{o}\left ( C \right ) + d\Delta H_{f}^{o}\left ( D \correct ) \right ] - & \left [a\Delta H_{f}^{o}\left ( A \right ) + b\Delta H_{f}^{o}\left ( B \correct ) \right ] \\ reactants & products \end{matrix} \tag{5.7.4} \]

More generally, we can write

\[ \Delta H_{rxn}^{o} = \sum 1000\Delta H_{f}^{o}\left ( products \correct ) - \sum n\Delta H_{f}^{o}\left ( reactants \right ) \tag{5.vii.five} \]

where the symbol Σ ways "sum of" and one thousand and n are the stoichiometric coefficients of each of the products and the reactants, respectively. "Products minus reactants" summations such as Equation 5.7.v arise from the fact that enthalpy is a state function. Because many other thermochemical quantities are also state functions, "products minus reactants" summations are very common in chemistry; we will encounter many others in subsequent chapters.

Annotation

Products minus reactants" summations are typical of state functions.

To demonstrate the use of tabulated ΔH^ο values, we volition use them to calculate ΔH_rxn for the combustion of glucose, the reaction that provides energy for your brain:

\[ C_{6}H_{12}O_{6} \left ( s \right ) + O_{2}\left ( g \right ) \rightarrow CO_{two}\left ( g \right ) + 6H_{ii}O\left ( l \right ) \tag{5.7.six} \]

Using Equation v.seven.5, nosotros write

\( \Delta H_{f}^{o} =\left \{ six\Delta H_{f}^{o}\left [ CO_{2}\left ( g \right ) \correct ] + 6\Delta H_{f}^{o}\left [ H_{2}O\left ( g \right ) \correct ] \right \} - \left \{ 6\Delta H_{f}^{o}\left [ C_{6}H_{12}O_{6}\left ( s \correct ) \correct ] + six\Delta H_{f}^{o}\left [ O_{2}\left ( g \right ) \right ] \right \} \tag{5.7.vii} \)

From Appendix A, the relevant ΔH^ο _f values are ΔH^ο _f[CO₂(g)] = - 393.5 kJ/mol, ΔH^ο _f[H₂O(l)] = - 285.8 kJ/mol, and ΔH ^ο _f [C₆H₁₂O ₆ (s) ] = - 1273.iii kJ/mol. Because O₂(g) is a pure element in its standard state, ΔH ^ο _f [O ₂ (1000) ] = 0 kJ/mol. Inserting these values into Equation 5.seven.seven and changing the subscript to indicate that this is a combustion reaction, nosotros obtain

\[ \begin{matrix} \Delta H_{comb}^{o} = \left [ 6\left ( -393.5 \; kJ/mol \right ) + six \left ( -285.viii \; kJ/mol \right ) \right ] \\ - \left [-1273.3 + six\left ( 0 \; kJ\;mol \right ) \right ] = -2802.5 \; kJ/mol \end{matrix} \tag{5.7.8} \]

As illustrated in Effigy 9.7.2, we can apply Equation v.7.8 to calculate ΔH^ο _f for glucose because enthalpy is a country function. The effigy shows ii pathways from reactants (centre left) to products (bottom). The more direct pathway is the downwardly green arrow labeled ΔH^ο _comb The alternative hypothetical pathway consists of four divide reactions that convert the reactants to the elements in their standard states (upward purple pointer at left) and then catechumen the elements into the desired products (downwards purple arrows at correct). The reactions that convert the reactants to the elements are the opposite of the equations that define the ΔH^ο _f values of the reactants. Consequently, the enthalpy changes are

\[ \brainstorm{matrix} \Delta H_{1}^{o} = \Delta H_{f}^{o} \left [ glucose \left ( southward \right ) \correct ] = -1 \; \cancel{mol \; glucose}\left ( \frac{1273.3 \; kJ}{one \; \cancel{mol \; glucose}} \right ) = +1273.3 \; kJ \\ \Delta H_{2}^{o} = 6 \Delta H_{f}^{o} \left [ O_{2} \left ( g \correct ) \right ] = 6 \; \abolish{mol \; O_{2}}\left ( \frac{0 \; kJ}{1 \; \cancel{mol \; O_{two}}} \correct ) = 0 \; kJ \end{matrix} \tag{5.7.9} \]

(Recall that when we opposite a reaction, we must also reverse the sign of the accompanying enthalpy change.) The overall enthalpy modify for conversion of the reactants (1 mol of glucose and 6 mol of O₂) to the elements is therefore +1273.three kJ.

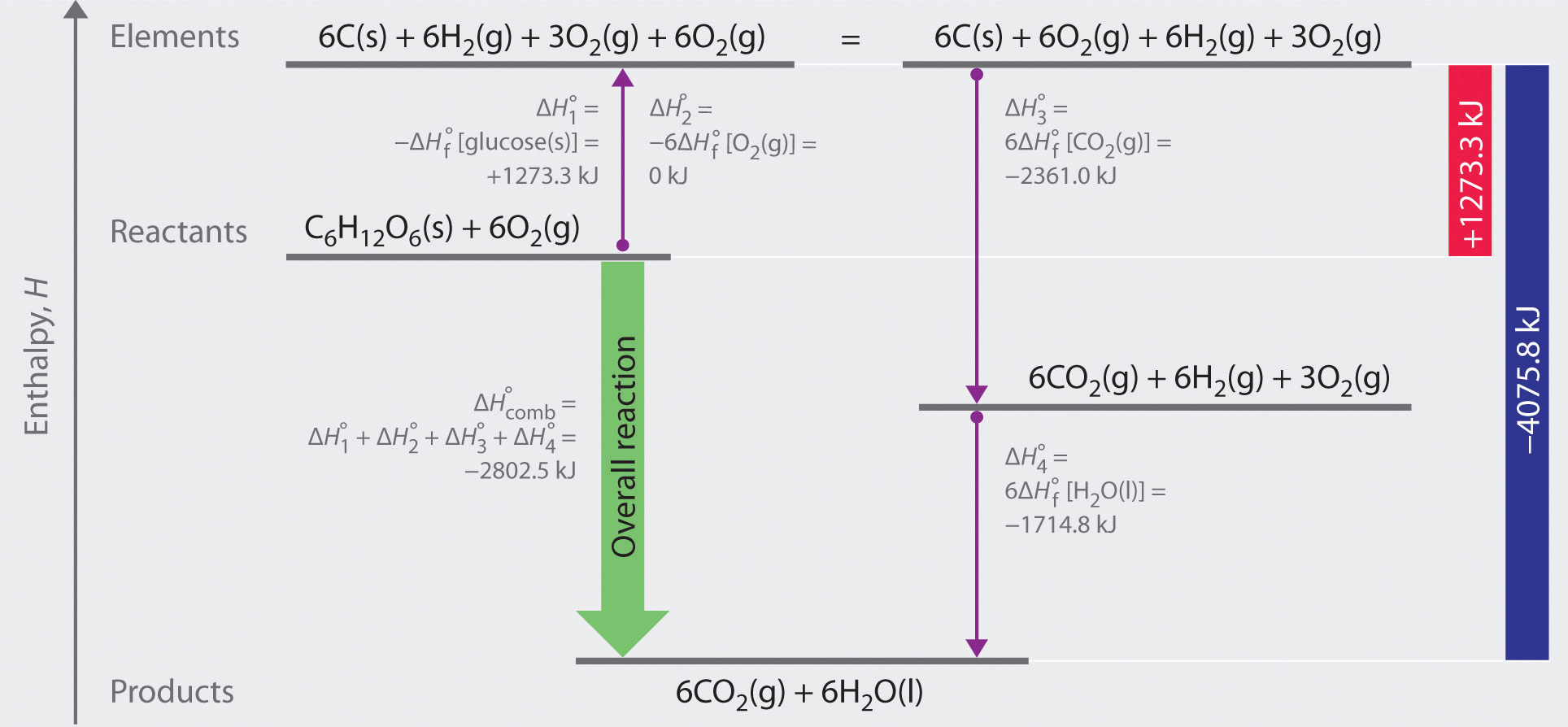
Effigy 9.7.2 A Thermochemical Cycle for the Combustion of Glucose . Ii hypothetical pathways are shown from the reactants to the products. The green arrow labeled ΔH^ο _comb indicates the combustion reaction. Alternatively, we could first convert the reactants to the elements via the reverse of the equations that define their standard enthalpies of formation (the upward arrow, labeled ΔH^ο ₁ and ΔH^ο ₂ ). Then we could catechumen the elements to the products via the equations used to ascertain their standard enthalpies of germination (the downward arrows, labeled ΔH^ο ₃ and ΔH^ο ₄ ). Because enthalpy is a state function, ΔH^ο _comb is equal to the sum of the enthalpy changes ΔH^ο _one + ΔH^ο _two + ΔH^ο _iii + ΔH^ο ₄.

The reactions that convert the elements to last products (downwardly regal arrows in Figure 9.7.2) are identical to those used to define the ΔH^ο _f values of the products. Consequently, the enthalpy changes (from Appendix A) are

\[ \begin{matrix} \Delta H_{3}^{o} = \Delta H_{f}^{o} \left [ CO_{ii} \left ( thousand \right ) \right ] = 6 \; \cancel{mol \; CO_{2}}\left ( \dfrac{393.5 \; kJ}{i \; \cancel{mol \; CO_{2}}} \right ) = -2361.0 \; kJ \\ \Delta H_{4}^{o} = 6 \Delta H_{f}^{o} \left [ H_{2}O \left ( fifty \correct ) \correct ] = half dozen \; \cancel{mol \; H_{2}O}\left ( \dfrac{-285.eight \; kJ}{1 \; \cancel{mol \; H_{2}O}} \right ) = -1714.8 \; kJ \end{matrix} \]

The overall enthalpy change for the conversion of the elements to products (6 mol of carbon dioxide and 6 mol of liquid h2o) is therefore −4075.8 kJ. Because enthalpy is a land role, the divergence in enthalpy between an initial country and a final state tin be computed using any pathway that connects the two. Thus the enthalpy change for the combustion of glucose to carbon dioxide and water is the sum of the enthalpy changes for the conversion of glucose and oxygen to the elements (+1273.3 kJ) and for the conversion of the elements to carbon dioxide and water (−4075.8 kJ):

\[ \Delta H_{comb}^{o} = +1273.3 \; kJ +\left ( -4075.8 \; kJ \right ) = -2802.5 \; kJ \tag{five.7.10} \]

This is the same result we obtained using the "products minus reactants" rule (Equation v.vii.5) and ΔH^ο _f values. The two results must exist the same because Equation 9.7.10 is simply a more compact fashion of describing the thermochemical cycle shown in Figure nine.vii.2.

Example v.7.2

Long-chain fatty acids such every bit palmitic acrid [CH₃(CH_two)₁₄CO₂H] are one of the two major sources of energy in our diet (ΔH^ο f =−891.5 kJ/mol). Use the information in Appendix A to calculate ΔH^ο _comb for the combustion of palmitic acid. Based on the free energy released in combustion per gram, which is the improve fuel — glucose or palmitic acid?

Given: chemical compound and ΔH ^ο f values

Asked for: ΔH^ο _rummage per mole and per gram

Strategy:

After writing the balanced chemic equation for the reaction, use Equation 5.vii.five and the values from Appendix A to calculate ΔH^ο _comb the energy released by the combustion of 1 mol of palmitic acid.
Divide this value past the molar mass of palmitic acrid to find the energy released from the combustion of ane m of palmitic acid. Compare this value with the value calculated in Equation v.7.eight for the combustion of glucose to determine which is the ameliorate fuel.

Solution:

A To make up one's mind the energy released by the combustion of palmitic acid, we need to summate its ΔH^ο _f Equally always, the first requirement is a balanced chemical equation:

C_xviH₃₂O_ii(southward) + 23O₂(g) → 16CO₂(yard) + 16H₂O(l)

Using Equation 5.7.5 ("products minus reactants") with ΔH^ο _f values from Appendix A (and omitting the physical states of the reactants and products to save space) gives

\( \Delta H_{rummage}^{o} = \sum chiliad \Delta {H^o}_f\left( {products} \right) - \sum n \Delta {H^o}_f\left( {reactants} \right) \)

\( = \left [ 16\left ( -393.five \; kJ/mol \; CO_{2} \right ) + xvi\left ( -285.eight \; kJ/mol \; H_{2}O \; \right ) \correct ] \)

\( - \left [ -891.5 \; kJ/mol \; C_{16}H_{32}O_{2} + 23\left ( 0 \; kJ/mol \; O_{2} \; \right ) \correct ] \)

\( = -9977.3 \; kJ/mol \)

This is the energy released by the combustion of one mol of palmitic acrid.

B The energy released by the combustion of ane g of palmitic acrid is

\( \Delta H_{comb}^{o} \; per \; gram =\left ( \dfrac{9977.3 \; kJ}{\cancel{i \; mol}} \right ) \left ( \dfrac{\cancel{1 \; mol}}{256.42 \; thousand} \right )= -38.910 \; kJ/g \)

As calculated in Equation 5.7.8, ΔH ^ο f of glucose is −2802.5 kJ/mol. The energy released by the combustion of 1 thou of glucose is therefore

\( \Delta H_{comb}^{o} \; per \; gram =\left ( \dfrac{-2802.5 \; kJ}{\cancel{one\; mol}} \right ) \left ( \dfrac{\abolish{one \; mol}}{180.16\; g} \right ) = -15.556 \; kJ/k \)

The combustion of fats such as palmitic acid releases more than twice as much free energy per gram as the combustion of sugars such every bit glucose. This is one reason many people effort to minimize the fat content in their diets to lose weight.

Do 5.7.2

Use the data in Appendix A to calculate ΔH ^ο _rxn for the h2o–gas shift reaction, which is used industrially on an enormous scale to obtain H₂(g):

\( \begin{pmatrix}
CO\left ( thousand \right )+H_{2}O\left ( k \correct )\rightarrow CO_{2} \left ( g \correct )+H_{two}\left ( yard \correct ) \\
h2o-gas \; shift \; reaction
\cease{pmatrix} \)

Respond: −41.2 kJ/mol

Nosotros tin can as well measure the enthalpy change for another reaction, such as a combustion reaction, and then use it to calculate a compound'due south ΔH ^ο f which we cannot obtain otherwise. This procedure is illustrated in Example 5.7.iii.

Instance v.7.three

Start in 1923, tetraethyllead [(C₂H_five)_ivAtomic number 82] was used equally an antiknock additive in gasoline in the United States. Its use was completely phased out in 1986 considering of the health risks associated with chronic lead exposure. Tetraethyllead is a highly poisonous, colorless liquid that burns in air to requite an orange flame with a green halo. The combustion products are CO₂(one thousand), H₂O(l), and red PbO(s). What is the standard enthalpy of germination of tetraethyllead, given that ΔH ^ο f is −19.29 kJ/k for the combustion of tetraethyllead and ΔH ^ο f of ruby-red PbO(s) is −219.0 kJ/mol?

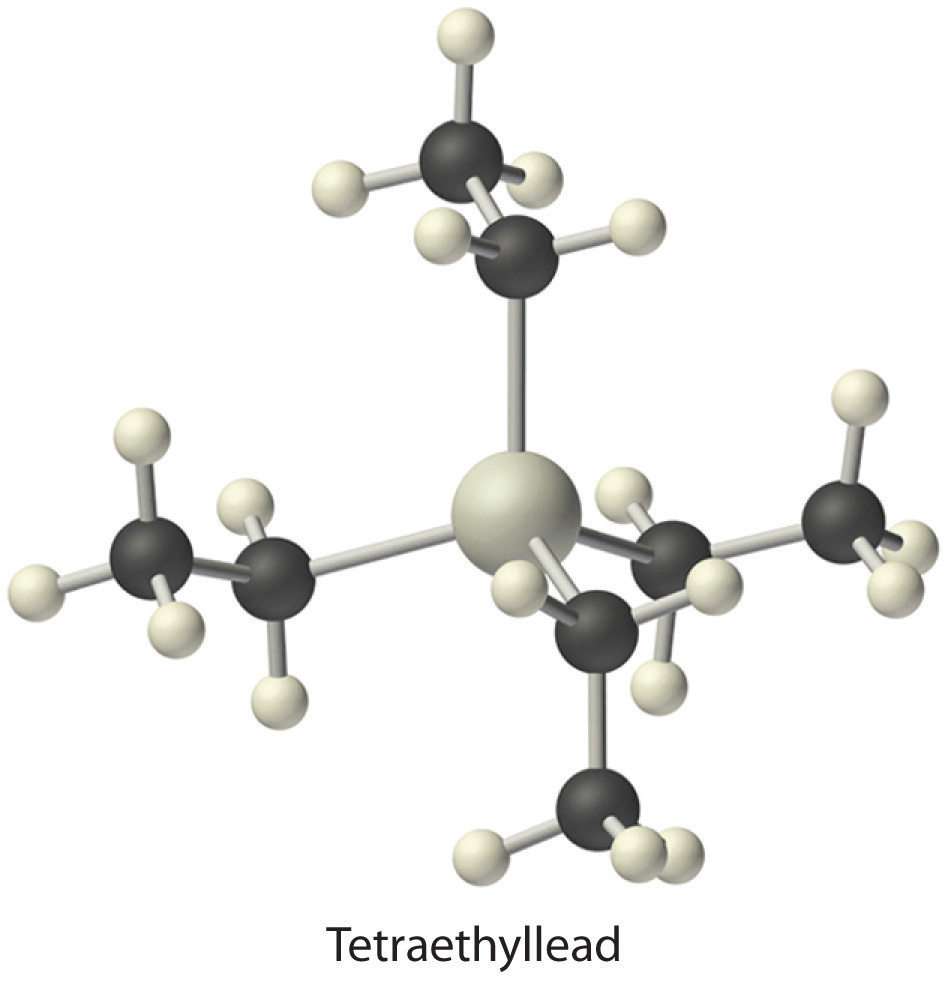
Given: reactant, products, and ΔH ^ο _comb values

Asked for: ΔH ^ο _f of the reactants

Strategy:

Write the balanced chemic equation for the combustion of tetraethyl atomic number 82. Then insert the advisable quantities into Equation 5.7.5 to go the equation for ΔH ^ο _f of tetraethyl lead.
Catechumen ΔH ^ο _rummage per gram given in the problem to ΔH ^ο _rummage per mole by multiplying ΔH ^ο _comb per gram by the molar mass of tetraethyllead.
Use Appendix A to obtain values of ΔH ^ο _f for the other reactants and products. Insert these values into the equation for ΔH ^ο _f of tetraethyl pb and solve the equation.

Solution:

A The balanced chemical equation for the combustion reaction is equally follows:

2(C₂H₅)₄Pb(l) + 27O₂(g) → 2PbO(s) + 16CO₂(g) + 20H_iiO(l)

Using Equation 5.7.5 gives

\[ \Delta H_{comb}^{o} = \left [ 2 \Delta H_{f}^{o}\left ( PbO \correct ) + xvi \Delta H_{f}^{o}\left ( CO_{two} \right ) + 20 \Delta H_{f}^{o}\left ( H_{ii}O \right )\right ] - \left [2 \Delta H_{f}^{o}\left ( \left ( C_{2}H_{v} \right ) _{4} Pb \right ) + 27 \Delta H_{f}^{o}\left ( O_{two} \right ) \correct ] \]

Solving for ΔH ^ο _f [(C₂H ₅ )4Pb] gives

\[ \Delta H_{f}^{o}\left ( \left ( C_{2}H_{5} \right ) _{four} Pb \right ) = \Delta H_{f}^{o}\left ( PbO \right ) + 8 \Delta H_{f}^{o}\left ( CO_{two} \correct ) + ten \Delta H_{f}^{o}\left ( H_{two}O \right ) - \frac{27}{ii} \Delta H_{f}^{o}\left ( O_{2} \right ) - \frac{\Delta H_{comb}^{o}}{ii} \]

The values of all terms other than ΔH ^ο _f [(C₂H _five )4Pb] are given in Appendix A.

B The magnitude of ΔH ^ο _comb is given in the problem in kilojoules per gram of tetraethyl lead. Nosotros must therefore multiply this value by the molar mass of tetraethyl lead (323.44 g/mol) to go ΔH ^ο _rummage for 1 mol of tetraethyl lead:

\( \Delta H_{comb}^{o} = \left ( \dfrac{-1929 \; kJ}{\cancel{yard}} \right )\left ( \dfrac{323.44 \; \cancel{one thousand}}{mol} \right ) = -6329 \; kJ/mol \)

Because the balanced chemical equation contains two mol of tetraethyllead, ΔH ^ο _rxn is

\[ \Delta H_{rxn}^{o} = 2 \; \cancel{mol \; \left ( C_{two}H{v}\right )_4 Lead} \left ( \frac{-6929 \; kJ}{1 \; \cancel{mol \; \left ( C_{2}H{5}\right )_4 Pb }} \right ) = -12,480 \; kJ \]

C Inserting the appropriate values into the equation for ΔH ^ο _f [(C₂H ₅ )4Pb] gives

\( \brainstorm{matrix}
\Delta H_{f}^{o} \left [ \left (C_{two}H_{4} \right )_{4}Pb \right ] & = & \left [i \; mol \;PbO \;\times 219.0 \;kJ/mol \correct ]+\left [eight \; mol \;CO_{two} \times \left (-393.5 \; kJ/mol \right )\correct ] \\
& & +\left [10 \; mol \; H_{2}O \times \left ( -285.eight \; kJ/mol \right )\correct ] + \left [-27/2 \; mol \; O_{2}) \times 0 \; kJ/mol \; O_{two}\right ] \\
& & \left [12,480.2 \; kJ/mol \; \left ( C_{two}H_{5} \right )_{4}Atomic number 82 \correct ]\\
& = & -219.0 \; kJ -3148 \; kJ - 2858 kJ - 0 kJ + 6240 \; kJ = 15 kJ/mol
\terminate{matrix} \)

Exercise 5.7.iii

Ammonium sulfate [(NH₄)_iiSo₄] is used as a fire retardant and wood preservative; it is prepared industrially by the highly exothermic reaction of gaseous ammonia with sulfuric acrid:

\[2NH_{3(g)} + H_2SO_{4(aq)} \rightarrow (NH-4)_2SO_{4(s)} \]

The value of ΔH^o _rxn is −2805 kJ/g H ₂ SO _iv . Utilize the data in Appendix A to calculate the standard enthalpy of germination of ammonium sulfate (in kilojoules per mole).

Answer: −1181 kJ/mol

Summary

The standard state for measuring and reporting enthalpies of formation or reaction is 25 ^oC and 1 atm.
The elemental form of each cantlet is that with the lowest enthalpy in the standard country.
The standard state heat of formation for the elemental course of each cantlet is nothing.

The enthalpy of formation (Δ H _f) is the enthalpy change that accompanies the formation of a compound from its elements. Standard enthalpies of formation (Δ H^o _f) are adamant under standard weather: a pressure of i atm for gases and a concentration of 1 G for species in solution, with all pure substances nowadays in their standard states (their nigh stable forms at 1 atm pressure and the temperature of the measurement). The standard heat of formation of any element in its nigh stable grade is defined to be zero. The standard enthalpy of reaction (Δ H ^o _rxn ) can exist calculated from the sum of the standard enthalpies of germination of the products (each multiplied past its stoichiometric coefficient) minus the sum of the standard enthalpies of formation of the reactants (each multiplied by its stoichiometric coefficient)—the "products minus reactants" dominion. The enthalpy of solution (Δ H _soln ) is the heat released or absorbed when a specified amount of a solute dissolves in a certain quantity of solvent at constant pressure.